
HISTORY OF MATHEMATICS
The area of study known as the history of mathematics is primarily an investigation into the origin of new discoveries in mathematics and, to a lesser extent, an investigation into the standard mathematical methods and notation of the past.
Before the modern age and the worldwide spread of knowledge, written examples of new mathematical developments have come to light only in a few locales. The most ancient mathematical texts available are Plimpton 322 (Babylonian mathematics ca. 1900 BC), the Moscow Mathematical Papyrus (Egyptian mathematics ca. 1850 BC), the Rhind Mathematical Papyrus (Egyptian mathematics ca. 1650 BC), and the Shulba Sutras (Indian mathematics ca. 800 BC). All of these texts concern the so-called Pythagorean theorem, which seems to be the most ancient and widespread mathematical development after basic arithmetic and geometry.
Egyptian and Babylonian mathematics were then further developed in Greek and Hellenistic mathematics, which is generally considered to be one of the most important for greatly expanding both the method and the subject matter of mathematics.[1] The mathematics developed in these ancient civilizations were then further developed and greatly expanded in Islamic mathematics. Many Greek and Arabic texts on mathematics were then translated into Latin in medieval Europe and further developed there.
One striking feature of the history of ancient and medieval mathematics is that bursts of mathematical development were often followed by centuries of stagnation. Beginning in Renaissance Italy in the 16th century, new mathematical developments, interacting with new scientific discoveries, were made at an ever increasing pace, and this continues to the present day.

EARLY MATHEMATICS
Long before the earliest written records, there are drawings that indicate some knowledge of elementary mathematics and of time measurement based on the stars. For example, paleontologists have discovered in a cave in South Africa, ochre rocks about 70,000 years old, adorned with scratched geometric patterns.[2] Also prehistoric artifacts discovered in Africa and France, dated between 35,000 and 20,000 years old,[3] suggest early attempts to quantify time.[4]
There is evidence that women devised counting to keep track of their menstrual cycles; 28 to 30 scratches on bone or stone, followed by a distinctive marker. Moreover, hunters and herders employed the concepts of one, two, and many, as well as the idea of none or zero, when considering herds of animals.[5][6]
The Ishango bone, found near the headwaters of the Nile river (northeastern Congo), may be as much as 20,000 years old. One common interpretation is that the bone is the earliest known demonstration[7] of sequences of prime numbers and of Ancient Egyptian multiplication. Predynastic Egyptians of the 5th millennium BC pictorially represented geometric spatial designs. It has been claimed that megalithic monuments in England and Scotland, dating from the 3rd millennium BC, incorporate geometric ideas such as circles, ellipses, and Pythagorean triples in their design.[8]
The earliest known mathematics in ancient India dates from 3000-2600 BC in the Indus Valley Civilization (Harappan civilization) of North India and Pakistan. This civilization developed a system of uniform weights and measures that used the decimal system, a surprisingly advanced brick technology which utilized ratios, streets laid out in perfect right angles, and a number of geometrical shapes and designs, including cuboids, barrels, cones, cylinders, and drawings of concentric and intersecting circles and triangles. Mathematical instruments included an accurate decimal ruler with small and precise subdivisions, a shell instrument that served as a compass to measure angles on plane surfaces or in horizon in multiples of 40–360 degrees, a shell instrument used to measure 8–12 whole sections of the horizon and sky, and an instrument for measuring the positions of stars for navigational purposes. The Indus script has not yet been deciphered; hence very little is known about the written forms of Harappan mathematics. Archeological evidence has led some to suspect that this civilization used a base 8 numeral system and had a value of π, the ratio of the length of the circumference of the circle to its diameter.[9]
The earliest extant Chinese mathematics dates from the Shang Dynasty (1600—1046 BC), and consists of numbers scratched on a tortoise shell [1] [2]. These numbers were represented by means of a decimal notation. For example, the number 123 is written (from top to bottom) as the symbol for 1 followed by the symbol for 100, then the symbol for 2 followed by the symbol for 10, then the symbol for 3. This was the most advanced number system in the world at the time, and allowed calculations to be carried out on the suan pan or (Chinese abacus). The date of the invention of the suan pan is not certain, but the earliest written mention dates from AD 190, in Xu Yue's Supplementary Notes on the Art of Figures.

ANCIENT INDIAN MATHEMATICS
Vedic mathematics began in the early Iron Age, with the Shatapatha Brahmana (c. 9th century BC), which approximates the value of π to 2 decimal places.[10], and the Sulba Sutras (c. 800-500 BC) were geometry texts that used irrational numbers, prime numbers, the rule of three and cube roots; computed the square root of 2 to five decimal places; gave the method for squaring the circle; solved linear equations and quadratic equations; developed Pythagorean triples algebraically and gave a statement and numerical proof of the Pythagorean theorem.
Pāṇini (c. 5th century BC) formulated the grammar rules for Sanskrit. His notation was similar to modern mathematical notation, and used metarules, transformations, and recursions with such sophistication that his grammar had the computing power equivalent to a Turing machine. Pingala (roughly 3rd-1st centuries BC) in his treatise of prosody uses a device corresponding to a binary numeral system. His discussion of the combinatorics of meters, corresponds to the binomial theorem. Pingala's work also contains the basic ideas of Fibonacci numbers (called mātrāmeru). The Brāhmī script was developed at least from the Maurya dynasty in the 4th century BC, with recent archeological evidence appearing to push back that date to around 600 BC. The Brahmi numerals date to the 3rd century BC.
Between 400 BC and AD 200, Jaina mathematicians began studying mathematics for the sole purpose of mathematics. They were the first to develop transfinite numbers, set theory, logarithms, fundamental laws of indices, cubic equations, quartic equations, sequences and progressions, permutations and combinations, squaring and extracting square roots, and finite and infinite powers. The Bakhshali Manuscript written between 200 BC and AD 200 included solutions of linear equations with up to five unknowns, the solution of the quadratic equation, arithmetic and geometric progressions, compound series, quadratic indeterminate equations, simultaneous equations, and the use of zero and negative numbers. Accurate computations for irrational numbers could be found, which includes computing square roots of numbers as large as a million to at least 11 decimal places.

GREEK HELLENISTIC MATHEMATICS
Greek mathematics refers to mathematics written in Greek between about 600 BCE and 450 CE.[13] Greek mathematicians lived in cities spread over the entire Eastern Mediterranean, from Italy to North Africa, but were united by culture and language. Greek mathematics of the period following Alexander the Great is sometimes called Hellenistic mathematics.
Greek mathematics was much more sophisticated than the mathematics that had been developed by earlier cultures. All surviving records of pre-Greek mathematics show the use of inductive reasoning, that is, repeated observations used to establish rules of thumb. Greek mathematicians, by contrast, used deductive reasoning. The Greeks used logic to derive conclusions from definitions and axioms.[14]
Greek mathematics is thought to have begun with Thales (c. 624—c.546 BC) and Pythagoras (c. 582—c. 507 BC). Although the extent of the influence is disputed, they were probably inspired by the ideas of Egypt, Mesopotamia and perhaps India. According to legend, Pythagoras travelled to Egypt to learn mathematics, geometry, and astronomy from Egyptian priests.
Thales used geometry to solve problems such as calculating the height of pyramids and the distance of ships from the shore. Pythagoras is credited with the first proof of the Pythagorean theorem, though the statement of the theorem has a long history.[15] In his commentary on Euclid, Proclus states that Pythagoras expressed the theorem that bears his name and constructed Pythagorean triples algebraically rather than geometrically. The Academy of Plato had the motto "let none unversed in geometry enter here".
The Pythagoreans discovered the existence of irrational numbers. Eudoxus (408 —c.355 BC) developed the method of exhaustion, a precursor of modern integration. Aristotle (384—c.322 BC) first wrote down the laws of logic. Euclid (c. 300 BC) is the earliest example of the format still used in mathematics today, definition, axiom, theorem, proof. He also studied conics. His book, Elements, was known to all educated people in the West until the middle of the 20th century.[16] In addition to the familiar theorems of geometry, such as the Pythagorean theorem, Elements includes a proof that the square root of two is irrational and that there are infinitely many prime numbers. The Sieve of Eratosthenes (ca. 230 BC) was used to discover prime numbers.
Archimedes (c.287—212 BC) of Syracuse used the method of exhaustion to calculate the area under the arc of a parabola with the summation of an infinite series, and gave remarkably accurate approximations of Pi.[17] He also defined the spiral bearing his name, formulas for the volumes of surfaces of revolution and an ingenious system for expressing very large numbers.
 CLASSICAL CHINESE MATHEMATICS
CLASSICAL CHINESE MATHEMATICSIn
China(212 BC), the Emperor
Qin Shi Huang (Shi Huang-ti) commanded that all books outside of Qin state to be burned. It was not universally obeyed, but as a consequence of this order little is known with certainty about ancient Chinese mathematics.
From the Western Zhou Dynasty (from 1046 BC), the oldest mathematical work to survive the book burning is the I Ching, which uses the 8 binary 3-tuples (trigrams) and 64 binary 6-tuples (hexagrams) for philosophical, mathematical, and/or mystical purposes. The binary tuples are composed of broken and solid lines, called yin 'female' and yang 'male' respectively (see King Wen sequence).
The oldest existent work on geometry in China comes from the philosophical Mohist canon of c. 330 BC, compiled by the followers of Mozi (470 BC-390 BC). The Mo Jing described various aspects of many fields associated with physical science, and provided a small wealth of information on mathematics as well.
After the book burning, the Han dynasty (202 BC–220 AD) produced works of mathematics which presumably expand on works that are now lost. The most important of these is The Nine Chapters on the Mathematical Art, the full title of which appeared by 179 AD, but existed in part under other titles beforehand. It consists of 246 word problems, involving agriculture, business, employment of geometry to figure height spans and dimension ratios for Chinese pagoda towers, engineering, surveying, and includes material on right triangles and π. It also made use of Cavalieri's principle on volume more than a thousand years before Cavalieri would propose it in the West. It created mathematical proof for Pythagoras' Pythagorean theorem, and mathematical formula for Gaussian elimination. The work was commented on by Liu Hui in the 3rd century AD.
In addition, the mathematical works of the Han astronomer and inventor Zhang Heng (78-139 AD) had a formulation for pi as well, which differed from Liu Hui's calculation. Zhang Heng used his formula of pi to find spherical volume. There was also the written work of the mathematician and music theorist Jing Fang (78–37 BC); by using the Pythagorean comma, Jing observed that 53 just fifths approximates to 31 octaves. This would later lead to the discovery of 53 equal temperament, and was not calculated precisely elsewhere until the German Nicholas Mercator did so in the 17th century.
The Chinese also made use of the complex combinatorial diagram known as the 'magic square and magic circles which was described in ancient times and perfected by Yang Hui (1238–1398 AD).
Zu Chongzhi (5th century) of the Southern and Northern Dynasties computed the value of π to seven decimal places, which remained the most accurate value of π for almost 1000 years.
In the thousand years following the Han dynasty, starting in the Tang dynasty and ending in the Song dynasty, Chinese mathematics thrived at a time when European mathematics did not exist[citation needed]. Developments first made in China[citation needed], and only much later known in the West, include negative numbers, the binomial theorem[citation needed], matrix methods for solving systems of linear equations[citation needed] and the Chinese remainder theorem. The Chinese also developed Pascal's triangle[citation needed] and the rule of three long before it was known in Europe. Besides Zu Chongzhi, some of the most important figures of Chinese mathematics during this period include Yi Xing, Shen Kuo, Qin Jiushao, Zhu Shijie, and others. The scientist Shen Kuo used problems involving calculus, trigonometry, metrology, permutations, and once computed the possible amount of terrain space that could be used with specific battle formations, as well as the longest possible military campaign given the amount of food carriers could bring for themselves and soldiers.
Even after European mathematics began to flourish during the Renaissance, European and Chinese mathematics were separate traditions, with significant Chinese mathematical output in decline, until the Jesuit missionaries such as Matteo Ricci carried mathematical ideas back and forth between the two cultures from the 16th to 18th centuries.

THE CLASSICAL INDIAN MATHEMATICS
The Surya Siddhanta (c. 400) introduced the trigonometric functions of sine, cosine, and inverse sine, and laid down rules to determine the true motions of the luminaries, which conforms to their actual positions in the sky. The cosmological time cycles explained in the text, which was copied from an earlier work, corresponds to an average sidereal year of 365.2563627 days, which is only 1.4 seconds longer than the modern value of 365.25636305 days. This work was translated to Arabic and Latin during the Middle Ages.
Aryabhata in 499 introduced the versine function, produced the first trigonometric tables of sine, developed techniques and algorithms of algebra, infinitesimals, differential equations, and obtained whole number solutions to linear equations by a method equivalent to the modern method, along with accurate astronomical calculations based on a heliocentric system of gravitation. An Arabic translation of his Aryabhatiya was available from the 8th century, followed by a Latin translation in the 13th century. He also computed the value of π to the fourth decimal place as 3.1416. Madhava later in the 14th century computed the value of π to the eleventh decimal place as 3.14159265359.
In the 7th century, Brahmagupta identified the Brahmagupta theorem, Brahmagupta's identity and Brahmagupta's formula, and for the first time, in Brahma-sphuta-siddhanta, he lucidly explained the use of zero as both a placeholder and decimal digit and explained the Hindu-Arabic numeral system. It was from a translation of this Indian text on mathematics (around 770) that Islamic mathematicians were introduced to this numeral system, which they adapted as Arabic numerals. Islamic scholars carried knowledge of this number system to Europe by the 12th century, and it has now displaced all older number systems throughout the world. In the 10th century, Halayudha's commentary on Pingala's work contains a study of the Fibonacci sequence and Pascal's triangle, and describes the formation of a matrix.
In the 12th century, Bhaskara first conceived differential calculus, along with the concepts of the derivative, differential coefficient and differentiation. He also stated Rolle's theorem (a special case of the mean value theorem), studied Pell's equation, and investigated the derivative of the sine function. From the 14th century, Madhava and other Kerala School mathematicians, further developed his ideas. They developed the concepts of mathematical analysis and floating point numbers, and concepts fundamental to the overall development of calculus, including the mean value theorem, term by term integration, the relationship of an area under a curve and its antiderivative or integral, tests of convergence, iterative methods for solutions of non-linear equations, and a number of infinite series, power series, Taylor series and trigonometric series. In the 16th century, Jyeshtadeva consolidated many of the Kerala School's developments and theorems in the Yuktibhasa, the world's first differential calculus text, which also introduced concepts of integral calculus. Mathematical progress in India became stagnant from the late 16th century onwards due to subsequent political turmoil.

ISLAMIC MATHEMATICS
The Islamic Arab Empire established across the Middle East, Central Asia, North Africa, Iberia, and in parts of India in the 8th century made significant contributions towards mathematics. Although most Islamic texts on mathematics were written in Arabic, they were not all written by Arabs, since much like the status of Greek in the Hellenistic world, Arabic was used as the written language of non-Arab scholars throughout the Islamic world at the time. Alongside Arabs, many important Islamic mathematicians were also Persians.
In the 9th century, Muḥammad ibn Mūsā al-Ḵwārizmī wrote several important books on the Hindu-Arabic numerals and on methods for solving equations. His book On the Calculation with Hindu Numerals, written about 825, along with the work of Al-Kindi, were instrumental in spreading Indian mathematics and Indian numerals to the West. The word algorithm is derived from the Latinization of his name, Algoritmi, and the word algebra from the title of one of his works, Al-Kitāb al-mukhtaṣar fī hīsāb al-ğabr wa’l-muqābala (The Compendious Book on Calculation by Completion and Balancing). Al-Khwarizmi is often called the "father of algebra", for his fundamental contributions to the field.[18] He gave an exhaustive explanation for the algebraic solution of quadratic equations with positive roots,[19] and he was the first to teach algebra in an elementary form and for its own sake.[20] He also introduced the fundamental method of "reduction" and "balancing", referring to the transposition of subtracted terms to the other side of an equation, that is, the cancellation of like terms on opposite sides of the equation. This is the operation which Al-Khwarizmi originally described as al-jabr.[21] His algebra was also no longer concerned "with a series of problems to be resolved, but an exposition which starts with primitive terms in which the combinations must give all possible prototypes for equations, which henceforward explicitly constitute the true object of study." He also studied an equation for its own sake and "in a generic manner, insofar as it does not simply emerge in the course of solving a problem, but is specifically called on to define an infinite class of problems."[22]
Further developments in algebra were made by Al-Karaji in his treatise al-Fakhri, where he extends the methodology to incorporate integer powers and integer roots of unknown quantities. The first known proof by mathematical induction appears in a book written by Al-Karaji around 1000 AD, who used it to prove the binomial theorem, Pascal's triangle, and the sum of integral cubes.[23] The historian of mathematics, F. Woepcke,[24] praised Al-Karaji for being "the first who introduced the theory of algebraic calculus." Also in the 10th century, Abul Wafa translated the works of Diophantus into Arabic and developed the tangent function. Ibn al-Haytham was the first mathematician to derive the formula for the sum of the fourth powers, using a method that is readily generalizable for determining the general formula for the sum of any integral powers. He performed an integration in order to find the volume of a paraboloid, and was able to generalize his result for the integrals of polynomials up to the fourth degree. He thus came close to finding a general formula for the integrals of polynomials, but he was not concerned with any polynomials higher than the fourth degree.[25]
In the late 11th century, Omar Khayyam wrote Discussions of the Difficulties in Euclid, a book about flaws in Euclid's Elements, especially the parallel postulate, and thus he laid the foundations for analytic geometry and non-Euclidean geometry. He was also the first to find the general geometric solution to cubic equations. He was also very influential in calendar reform. In the late 12th century, Sharaf al-Dīn al-Tūsī introduced the concept of a function,[26] and he was the first to discover the derivative of cubic polynomials.[27] His Treatise on Equations developed concepts related to differential calculus, such as the derivative function and the maxima and minima of curves, in order to solve cubic equations which may not have positive solutions.[28] In the 13th century, Nasir al-Din Tusi (Nasireddin) made advances in spherical trigonometry. He also wrote influential work on Euclid's parallel postulate. In the 15th century, Ghiyath al-Kashi computed the value of π to the 16th decimal place. Kashi also had an algorithm for calculating nth roots, which was a special case of the methods given many centuries later by Ruffini and Horner. Other notable Muslim mathematicians included al-Samawal, Abu'l-Hasan al-Uqlidisi, Jamshid al-Kashi, Thabit ibn Qurra, Abu Kamil and Abu Sahl al-Kuhi.
Other achievements of Muslim mathematicians during this period include the development of algebra and algorithms (see Muhammad ibn Mūsā al-Khwārizmī), the development of spherical trigonometry,[29] the addition of the decimal point notation to the Arabic numerals, the discovery of all the modern trigonometric functions besides sine, al-Kindi's introduction of cryptanalysis and frequency analysis, the development of analytic geometry by Ibn al-Haytham, the beginning of algebraic geometry by Omar Khayyam, the first refutations of Euclidean geometry and the parallel postulate by Nasīr al-Dīn al-Tūsī, the first attempt at a non-Euclidean geometry by Sadr al-Din, the development of an algebraic notation by al-Qalasādī,[30] and many other advances in algebra, arithmetic, calculus, cryptography, geometry, number theory and trigonometry. During the time of the Ottoman Empire from the 15th century, the development of Islamic mathematics became stagnant.
EARLY MODERN EUROPEAN MATHEMATICS
In Europe at the dawn of the Renaissance, mathematics was still limited by the cumbersome notation using Roman numerals and expressing relationships using words, rather than symbols: there was no plus sign, no equal sign, and no use of x as an unknown.[citation needed]
In 16th century European mathematicians began to make advances without precedent anywhere in the world, so far as is known today. The first of these was the general solution of cubic equations, generally credited to Scipione del Ferro circa 1510, but first published by Johannes Petreius in Nuremberg in Gerolamo Cardano's Ars magna, which also included the solution of the general quartic equation from Cardano's student Lodovico Ferrari .
From this point on, mathematical developments came swiftly, contributing to and benefiting from contemporary advances in the physical sciences. This progress was greatly aided by advances in printing. The earliest mathematical books printed were Peurbach's Theoricae nova planetarum 1472 followed by a book on commercial arithmetic, the 1478 Treviso Arithmetic and then the first real mathematics book Euclid's Elements printed and published by Ratdolt 1482.
Driven by the demands of navigation and the growing need for accurate maps of large areas, trigonometry grew to be a major branch of mathematics. Bartholomaeus Pitiscus was the first to use the word, publishing his Trigonometria in 1595. Regiomontanus' table of sines and cosines was published in 1533.[42]
By century's end, thanks to Regiomontanus (1436—1476) and François Vieta (1540—1603), among others, mathematics was written using Hindu-Arabic numerals and in a form not too different from the notation used today.
17TH CENTURY
The 17th century saw an unprecedented explosion of mathematical and scientific ideas across Europe. Galileo, an Italian, observed the moons of Jupiter in orbit about that planet, using a telescope based on a toy imported from Holland. Tycho Brahe, a Dane, had gathered an enormous quantity of mathematical data describing the positions of the planets in the sky. His student, Johannes Kepler, a German, began to work with this data. In part because he wanted to help Kepler in his calculations, John Napier, in Scotland, was the first to investigate natural logarithms. Kepler succeeded in formulating mathematical laws of planetary motion. The analytic geometry developed by René Descartes (1596-1650), a French mathematician and philosopher, allowed those orbits to be plotted on a graph, in Cartesian coordinates. Building on earlier work by many mathematicians, Isaac Newton, an Englishman, discovered the laws of physics explaining Kepler's Laws, and brought together the concepts now known as calculus. Independently, Gottfried Wilhelm Leibniz, in Germany, developed calculus and much of the calculus notation still in use today. Science and mathematics had become an international endeavor, which would soon spread over the entire world.[43]
In addition to the application of mathematics to the studies of the heavens, applied mathematics began to expand into new areas, with the correspondence of Pierre de Fermat and Blaise Pascal. Pascal and Fermat set the groundwork for the investigations of probability theory and the corresponding rules of combinatorics in their discussions over a game of gambling. Pascal, with his wager, attempted to use the newly developing probability theory to argue for a life devoted to religion, on the grounds that even if the probability of success was small, the rewards were infinite. In some sense, this foreshadowed the development of utility theory in the 18th-19th century.

18TH CENTURY
As we have seen, knowledge of the natural numbers, 1, 2, 3,..., as preserved in monolithic structures, is older than any surviving written text. The earliest civilizations -- in Mesopotamia, Egypt, India and China -- knew arithmetic.
One way to view the development of the various number systems of modern mathematics is to see new numbers studied and investigated to answer questions about arithmetic performed on older numbers. In prehistoric times, fractions answered the question: what number, when multiplied by 3, gives the answer 1? In India and China, and much later in Germany, negative numbers were developed to answer the question: what do you get when you subtract a larger number from a smaller?
Another natural question is: what kind of a number is the square root of two? The Greeks knew that it was not a fraction, and this question may have played a role in the development of continued fractions. But a better answer came with the invention of decimals, developed by John Napier (1550 - 1617) and perfected later by Simon Stevin. Using decimals, and an idea that anticipated the concept of the limit, Napier also studied a new constant, which Leonhard Euler (1707 - 1783) named e.
Euler was very influential in the standardization of other mathematical terms and notations. He named the square root of minus 1 with the symbol i. He also popularized the use of the Greek letter π to stand for the ratio of a circle's circumference to its diameter. He then derived one of the most remarkable identities in all of mathematics:
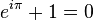 (see Euler's Identity.)
(see Euler's Identity.)

19TH CENTURY
Throughout the 19th century mathematics became increasingly abstract. In the 19th century lived Carl Friedrich Gauss (1777–1855). Leaving aside his many contributions to science, in pure mathematics he did revolutionary work on functions of complex variables, in geometry, and on the convergence of series. He gave the first satisfactory proofs of the fundamental theorem of algebra and of the quadratic reciprocity law.
This century saw the development of the two forms of non-Euclidean geometry, where the parallel postulate of Euclidean geometry no longer holds. The Russian mathematician Nikolai Ivanovich Lobachevsky and his rival, the Hungarian mathematician Janos Bolyai, independently discovered hyperbolic geometry, where uniqueness of parallels no longer holds. In this geometry the sum of angles in a triangle add up to less than 180°. Elliptic geometry was developed later in the 19th century by the German mathematician Bernhard Riemann; here no parallel can be found and the angles in a triangle add up to more than 180°. Riemann also developed Riemannian geometry, which unifies and vastly generalizes the three types of geometry, and he defined the concept of a manifold, which generalize the ideas of curves and surfaces.
The 19th century saw the beginning of a great deal of abstract algebra. William Rowan Hamilton in Ireland developed noncommutative algebra. The British mathematician George Boole devised an algebra that soon evolved into what is now called Boolean algebra, in which the only numbers were 0 and 1 and in which, famously, 1 + 1 = 1. Boolean algebra is the starting point of mathematical logic and has important applications in computer science.
Augustin-Louis Cauchy, Bernhard Riemann, and Karl Weierstrass reformulated the calculus in a more rigorous fashion.
Also, for the first time, the limits of mathematics were explored. Niels Henrik Abel, a Norwegian, and Évariste Galois, a Frenchman, proved that there is no general algebraic method for solving polynomial equations of degree greater than four. Other 19th century mathematicians utilized this in their proofs that straightedge and compass alone are not sufficient to trisect an arbitrary angle, to construct the side of a cube twice the volume of a given cube, nor to construct a square equal in area to a given circle. Mathematicians had vainly attempted to solve all of these problems since the time of the ancient Greeks.
Abel and Galois's investigations into the solutions of various polynomial equations laid the groundwork for further developments of group theory, and the associated fields of abstract algebra. In the 20th century physicists and other scientists have seen group theory as the ideal way to study symmetry.
In the later 19th century, Georg Cantor invented set theory, which enabled the rigorous treatment of the notion of infinity and has become the common language of nearly all mathematics. Cantor's set theory, and the rise of mathematical logic in the hands of Peano, L. E. J. Brouwer, David Hilbert, Bertrand Russell, and A.N. Whitehead, initiated a long running debate on the foundations of mathematics.
The 19th century saw the founding of a number of national mathematical societies: the London Mathematical Society in 1865, the Société Mathématique de France in 1872, the Circolo Mathematico di Palermo in 1884, the Edinburgh Mathematical Society in 1883, and the American Mathematical Society in 1888.

THW 20TH CENTURY
The 20th century saw mathematics become a major profession. Every year, thousands of new Ph.D.s in mathematics are awarded, and jobs are available in both teaching and industry. In earlier centuries, there were few creative mathematicians in the world at any one time. For the most part, mathematicians were either born to wealth, like Napier, or supported by wealthy patrons, like Gauss. A few, like Fourier, derived meager livelihoods from teaching in universities. Niels Henrik Abel, unable to obtain a position, died in poverty of malnutrition and tuberculosis at the age of twenty-six.
During the 20th century, the body of known mathematics grew at an exponential rate, so that this section will mention only a few of the most profound discoveries. In a 1900 speech to the International Congress of Mathematicians, David Hilbert set out a list of 23 unsolved problems in mathematics. These problems, spanning many areas of mathematics, formed a central focus for much of 20th century mathematics. Today, 10 have been solved, 7 are partially solved, and 2 are still open. The remaining 4 are too loosely formulated to be stated as solved or not.
Famous historical conjectures were finally proved. In 1976, Wolfgang Haken and Kenneth Appel used a computer to prove the four color theorem. Andrew Wiles, building on the work of others, proved Fermat's Last Theorem in 1995. Paul Cohen and Kurt Gödel proved that the continuum hypothesis is independent of (could neither be proved nor disproved from) the standard axioms of set theory.
Mathematical collaborations of unprecedented size and scope took place. A famous example is the classification of finite simple groups (also called the "enormous theorem"), whose proof between 1955 and 1983 required 500-odd journal articles by about 100 authors, and filling tens of thousands of pages. A group of French mathematicians, including Jean Dieudonné and André Weil, publishing under the pseudonym "Nicolas Bourbaki," attempted to exposit all of known mathematics as a coherent rigorous whole. The resulting several dozen volumes has had a controversial influence on mathematical education.[44]
Entire new areas of mathematics such as mathematical logic, topology, complexity theory, and game theory changed the kinds of questions that could be answered by mathematical methods.
At the same time, deep discoveries were made about the limitations to mathematics. In 1929 and 1930, it was discovered the truth or falsity of all statements formulated about the natural numbers plus one of addition and multiplication, was decidable, i.e., could be determined by algorithm. In 1931, Kurt Gödel found that this was not the case for the natural numbers plus both addition and multiplication; this system, known as Peano arithmetic, was in fact incompletable. (Peano arithmetic is adequate for a good deal of number theory, including the notion of prime number.) A consequence of Gödel's two incompleteness theorems is that in any mathematical system that includes Peano arithmetic (including all of analysis and geometry), truth necessarily outruns proof; there are true statements that cannot be proved within the system. Hence mathematics cannot be reduced to mathematical logic, and David Hilbert's dream of making all of mathematics complete and consistent died.
One of the more colorful figures in 20th century mathematics was Srinivasa Aiyangar Ramanujan (1887–1920) who, despite being largely self-educated, conjectured or proved over 3000 theorems, including properties of highly composite numbers, the partition function and its asymptotics, and mock theta functions. He also made major discoveries in the areas of gamma functions, modular forms, divergent series, hypergeometric series and prime number theory.














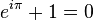 (see
(see 
